[Next] [Previous] [Up]
Problem # 10
Submitted by Richard Statman
Date: 1993
Statement. Do uniform universal generators exist?
[PRINT this PROBLEM]
A term  is a uniform universal generator iff there is a non-trivial
context
is a uniform universal generator iff there is a non-trivial
context ![C[*]](opltlca127x.png) such that
such that ![M ↠ C[N ]](opltlca128x.png) for each closed term
for each closed term 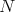 . The above
question was first stated in [Statman, 1993], and it is open for either CL (with
weak reduction) or lambda-calculus (with
. The above
question was first stated in [Statman, 1993], and it is open for either CL (with
weak reduction) or lambda-calculus (with 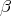 - or
- or  -reduction). If the context
-reduction). If the context
![C[ *]](opltlca132x.png) is required to have the form
is required to have the form 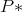 , so
, so ![C[N ]](opltlca134x.png) is
is 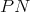 , then it can be
shown [Statman, 1993] that uniform universal generators do not exist for
combinatory logic.
, then it can be
shown [Statman, 1993] that uniform universal generators do not exist for
combinatory logic.
References for # 10
[Statman, 1993] Statman, R. (1993). Some examples of non-existent
combinators. Theoretical Computer Science, 121:441–448.
[Next] [Previous] [Up]
Last modified: July 21, 2014
 is a uniform universal generator iff there is a non-trivial
context
is a uniform universal generator iff there is a non-trivial
context ![C[*]](opltlca127x.png) such that
such that ![M ↠ C[N ]](opltlca128x.png) for each closed term
for each closed term 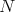 . The above
question was first stated in [Statman, 1993], and it is open for either CL (with
weak reduction) or lambda-calculus (with
. The above
question was first stated in [Statman, 1993], and it is open for either CL (with
weak reduction) or lambda-calculus (with 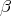 - or
- or  -reduction). If the context
-reduction). If the context
![C[ *]](opltlca132x.png) is required to have the form
is required to have the form 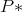 , so
, so ![C[N ]](opltlca134x.png) is
is 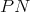 , then it can be
shown [Statman, 1993] that uniform universal generators do not exist for
combinatory logic.
, then it can be
shown [Statman, 1993] that uniform universal generators do not exist for
combinatory logic.